Abstract

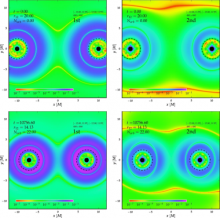
We construct a fully-analytic, general relativistic, non-spinning black hole binary spacetime that approximately solves the vacuum Einstein equations everywhere in space and time for black holes sufficiently well-separated. The metric is constructed by asymptotically matching perturbed Schwarzschild metrics near each black hole to a two-body post-Newtonian metric far from them, and a two-body post-Minkowskian metric farther still. Asymptotic matching is done without linearizing about a particular time slice, and thus it is valid dynamically and for all times, provided the binary is sufficiently well-separated. This approximate global metric can be used for long dynamical evolutions of relativistic magnetohydrodynamical, circumbinary disks around inspiraling supermassive black holes to study a variety of phenomena.